INTRODUCTION
Trigonometry (from Greek trigōnon "triangle" + metron "measure"[1] or from Sanskrit trikon "triangle" + miti "measurement" = trikonmiti[2]) is a branch of mathematicsthat studies triangles and the relationships between their sides and the angles between the sides. Trigonometry defines the trigonometric functions, which describe those relationships and have applicability to cyclical phenomena, such as waves. The field evolved during the third century BC as a branch of geometry used extensively for astronomical studies.[3]
Trigonometry is usually taught in middle and secondary schools either as a separate course or as part of a precalculus curriculum. It has applications in both pure mathematics and applied mathematics, where it is essential in many branches of science and technology. A branch of trigonometry, called spherical trigonometry, studies triangles on spheres, and is important in astronomy and navigation.
HISTORY
Ancient Egyptian and Babylonian mathematicians lacked the concept of an angle measure, but they studied the ratios of the sides of similar triangles and discovered some properties of these ratios. The ancient Greeks transformed trigonometry into an ordered science.[5]
Ancient Greek mathematicians such as Euclid and Archimedes studied the properties of the chord of an angle and proved theorems that are equivalent to modern trigonometric formulae, although they presented them geometrically rather than algebraically. Claudius Ptolemy expanded upon Hipparchus' Chords in a Circle in hisAlmagest.[6] The modern sine function was first defined in the Surya Siddhanta, and its properties were further documented by the 5th century Indian mathematicianand astronomer Aryabhata.[7] These Greek and Indian works were translated and expanded by medieval Islamic mathematicians. By the 10th century, Islamic mathematicians were using all six trigonometric functions, had tabulated their values, and were applying them to problems in spherical geometry. At about the same time, Chinese mathematicians developed trigonometry independently, although it was not a major field of study for them. Knowledge of trigonometric functions and methods reached Europe via Latin translations of the works of Persian and Arabic astronomers such as Al Battani and Nasir al-Din al-Tusi.[8] One of the earliest works on trigonometry by a European mathematician is De Triangulis by the 15th century German mathematician Regiomontanus. Trigonometry was still so little known in 16th century Europe that Nicolaus Copernicus devoted two chapters of De revolutionibus orbium coelestium to explaining its basic concepts.
Driven by the demands of navigation and the growing need for accurate maps of large areas, trigonometry grew to be a major branch of mathematics.[9]Bartholomaeus Pitiscus was the first to use the word, publishing his Trigonometria in 1595.[10] Gemma Frisius described for the first time the method of triangulationstill used today in surveying. It was Leonhard Euler who fully incorporated complex numbers into trigonometry. The works of James Gregory in the 17th century andColin Maclaurin in the 18th century were influential in the development of trigonometric series.[11] Also in the 18th century, Brook Taylor defined the general Taylor series.[12]
Applications of trigonometry
There are an enormous number of uses of trigonometry and trigonometric functions. For instance, the technique of triangulation is used in astronomy to measure the distance to nearby stars, in geography to measure distances between landmarks, and in satellite navigation systems. The sine and cosine functions are fundamental to the theory of periodic functions such as those that describe sound and light waves.
Fields that use trigonometry or trigonometric functions include astronomy (especially for locating apparent positions of celestial objects, in which spherical trigonometry is essential) and hence navigation (on the oceans, in aircraft, and in space), music theory, acoustics, optics, analysis of financial markets,electronics, probability theory, statistics, biology, medical imaging (CAT scans and ultrasound), pharmacy, chemistry, number theory (and hence cryptology),seismology, meteorology, oceanography, many physical sciences, land surveying and geodesy, architecture, phonetics, economics, electrical engineering,mechanical engineering, civil engineering, computer graphics, cartography, crystallography and game development.
Trigonometric Functions
- Sin, Cos, Tan Functions
- Cosec, Sec and Cot Functions
Sin, Cos and Tan Functions
The trigonometric functions of angles are the ratios of the various sides of a triangle. Consider a right-angled triangle ABC as shown in the figure below.
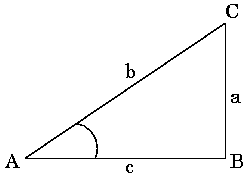
The following terminology is useful.
- Hypotenuse: The side opposite to the right angle in a triangle is called the hypotenuse. Here the side AC is the hypotenuse.
- Opposite Side: The side opposite to the angle in consideration is called the opposite side. So, if we are considering angle A, then the opposite side is CB.
- Base: The third side of the triangle, which is one of the arms of the angle under consideration, is called the base. If A is the angle under consideration, then the side AB is the base.
For angle A (sometimes referred to as angle CAB), the following fundamental trigonometric functions can be defined.
Sine of A = sin A = Opposite Side / Hypotenuse = CB/CA = a/b
Cosine of A = cos A = Base / Hypotenuse = AB/CA = c/b
Tangent of A = tan A = Opposite Side / Base = CB/AB = a/c
From the definitions, it can be seen that tan A = sin A / cos A.Cosec, Sec and Cot Functions
A few more useful functions can be defined.
Cosecant of A = cosec A = 1 / sin A = b/a
Secant of A = sec A = 1 / cos A = b/c
Cotangent of A = cot A = 1 / tan A = c/a
From the definitions, it can be seen that cot A = cosec A / sec A
|
From the definitions, it can be seen that tan A = sin A / cos A.
Cosecant of A = cosec A = 1 / sin A = b/a |
Secant of A = sec A = 1 / cos A = b/c |
Cotangent of A = cot A = 1 / tan A = c/a |
Values of Trigonometric Functions for Common Angles
It is useful to know the values of the trigonometric functions for certain common angles. The values of the trigonometric functions for angles 0, 30o, 45o, 60o and 90oare given in the table below.| Angle A | |||||
| sin A | |||||
| cos A | |||||
| tan A |
It is possible to obtain the values in the above table for 0, 45o and 90o by using the definitions from Trigonometry Module 1.
The values in the above table can be easily remembered using the following mnemonic. The values of sin 0, sin 30o, sin 45o, sin 60o and sin 90o are simply given by the square roots of 0/4, 1/4, 2/4, 3/4 and 4/4. The values of cos 0, cos 30o, cos 45o, cos 60o and cos 90o are given by the square roots of 4/4, 3/4, 2/4, 1/4 and 0/4. Obviously, the values of tan for any angle are obtained by dividing the sine value by the cosine value.
Important Trigonometric Identities
Some important trigonometric identities relating functions of a single angle (say, A) are given below.
sin2 A + cos2 A = 1 | ... (1) |
| 1 + tan2 A = sec2 A | ... (2) |
| 1+ cot2 A = cosec2 A | ... (3) |
These identities are useful in simplification and solution of problems. Their proofs are given below.
- Based on the right-angled triangle in the figure alongside, sin A = a / b and cos A = c / b.
Therefore sin2 A + cos2 A = (a2 + c2)/b2.
By Pythagoras Theorem, a2 + c2 = b2 in a right-angled triangle.
Thus sin2 A + cos2 A = 1 and equation (1) is proved.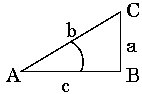
- On dividing both sides of equation (1) by cos2 A,
we obtain tan2 A + 1 = 1 / cos2 A = sec2 A. Equation (2) is proved.
- On dividing both sides of equation (1) by sin2 A,
we obtain 1 + cot2 A = 1/sin2 A = cosec2 A. Equation (3) is proved.Cofunction Property
The cofunction property is very important and is given below.
sin (90° − A) = cos A ... (4) cos (90° − A) = sin A ... (5) tan (90° − A) = cot A ... (6)
The cofunction property is easy to prove by starting with the fact that the sum of the three angles of a triangle is always 180o, i.e., A + B + C = 180o. For a right-angled triangle, angle B is 90o and A + C = 90o. On using 90o - C = A, the following are readily obtained: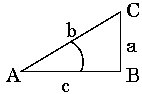
- sin (90o - A) = sin C = c / b = cos A
- cos (90o - A) = cos C = a / b = sin A
- tan (90o - A)
COMMON FORMULAS
Certain equations involving trigonometric functions are true for all angles and are known as trigonometric identities. Some identities equate an expression to a different expression involving the same angles. These are listed in List of trigonometric identities. Triangle identities that relate the sides and angles of a given triangle are listed below.In the following identities, A, B and C are the angles of a triangle and a, b and c are the lengths of sides of the triangle opposite the respective angles.
Law of sines
The law of sines (also known as the "sine rule") for an arbitrary triangle states:where R is the radius of the circumscribed circle of the triangle:Another law involving sines can be used to calculate the area of a triangle. Given two sides and the angle between the sides, the area of the triangle is:Law of cosines
The law of cosines (known as the cosine formula, or the "cos rule") is an extension of the Pythagorean theorem to arbitrary triangles:or equivalently:Law of tangents
The law of tangents:
Euler's formulaEuler's formula, which states that eix = cosx + isinx, produces the following analytical identities for sine, cosine, and tangent in terms of 'e' and the imaginary unit 'i' :
TRIGONOMETRY - MEASURE OF AN ANGLE
Any real number may be interpreted as the radian measure of an angle as follows: If
may be interpreted as the radian measure of an angle as follows: If  , think of wrapping a length
, think of wrapping a length  of string around the standard unit circle C in the plane, with initial point P(1,0), and proceeding counterclockwise around the circle; do the same if
of string around the standard unit circle C in the plane, with initial point P(1,0), and proceeding counterclockwise around the circle; do the same if  , but wrap the string clockwise around the circle. This process is described in Figure 1 below.
, but wrap the string clockwise around the circle. This process is described in Figure 1 below.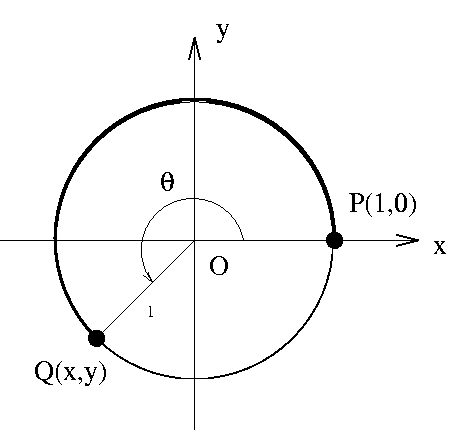
Figure 1 If Q(x,y) is the point on the circle where the string ends, we may think of as being an angle by associating to it the central angle with vertex O(0,0) and sides passing through the points P and Q. If instead of wrapping a length s of string around the unit circle, we decide to wrap it around a circle of radius R, the angle
as being an angle by associating to it the central angle with vertex O(0,0) and sides passing through the points P and Q. If instead of wrapping a length s of string around the unit circle, we decide to wrap it around a circle of radius R, the angle  (in radians) generated in the process will satisfy the following relation:Observe that the length s of string gives the measure of the angle
(in radians) generated in the process will satisfy the following relation:Observe that the length s of string gives the measure of the angle only when R=1.As a matter of common practice and convenience, it is useful to measure angles in degrees, which are defined by partitioning one whole revolution into 360 equal parts, each of which is then called one degree. In this way, one whole revolution around the unit circle measures
only when R=1.As a matter of common practice and convenience, it is useful to measure angles in degrees, which are defined by partitioning one whole revolution into 360 equal parts, each of which is then called one degree. In this way, one whole revolution around the unit circle measures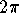 radians and also 360 degrees (or
radians and also 360 degrees (or  ), that is:Each degree may be further subdivided into 60 parts, called minutes, and in turn each minute may be subdivided into another 60 parts, called seconds:
), that is:Each degree may be further subdivided into 60 parts, called minutes, and in turn each minute may be subdivided into another 60 parts, called seconds:
EXAMPLE 1 Express the angle in Degree-Minute-Second (DMS) notation.Solution: We use Equation 3 to convert a fraction of a degree into minutes and a fraction of a minute into seconds:
in Degree-Minute-Second (DMS) notation.Solution: We use Equation 3 to convert a fraction of a degree into minutes and a fraction of a minute into seconds: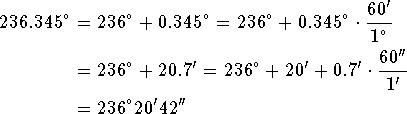
Therefore, .
.
The magic identity
Trigonometry is the art of doing algebra over the circle. So it is a mixture of algebra and geometry. The sine and cosine functions are just the coordinates of a point on the unit circle. This implies the most fundamental formula in trigonometry (which we will call here the magic identity)
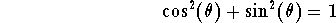 where
where is any real number (of course
is any real number (of course  measures an angle).Example. Show that
measures an angle).Example. Show that Answer. By definitions of the trigonometric functions we have
Answer. By definitions of the trigonometric functions we have Hence we have
Hence we have Using the magic identity we get
Using the magic identity we get This completes our proof.Remark. the above formula is fundamental in many ways. For example, it is very useful in techniques of integration.Example. Simplify the expression
This completes our proof.Remark. the above formula is fundamental in many ways. For example, it is very useful in techniques of integration.Example. Simplify the expression Answer. We have by definition of the trigonometric functions
Answer. We have by definition of the trigonometric functions Hence
Hence Using the magic identity we get
Using the magic identity we get Putting stuff together we get
Putting stuff together we get This gives
This gives Using the magic identity we get
Using the magic identity we get Therefore we have
Therefore we have
The Addition Formulas
The fundamental identities are very important for the analysis of trigonometric expressions and functions but they are a direct result of the intimate relation between trigonometry and geometry. The power behind the algebraic nature of trigonometry is hidden and can be measured only with the addition formulas
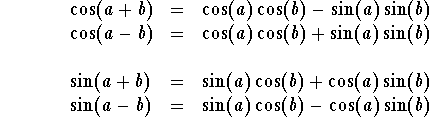 and
and Of course, we used the fact that
Of course, we used the fact that
Example. verify the identity
Answer. We have which gives
which gives But
But and since
and since and
and , we get finally
, we get finally
Remark. In general it is good to check whether the given formula is correct. One way to do that is to substitute some numbers for the variables. For example, if we take a=b = 0, we get or we may take
or we may take . In this case we have
. In this case we have
Example. Find the exact value of
Answer. We have Hence, using the additions formulas for the cosine function we get
Hence, using the additions formulas for the cosine function we get Since
Since we get
we get
Example. Find the exact value for
Answer. We have Since
Since we get
we get Finally we have
Finally we have
Remark. Using the addition formulas, we generate the following identities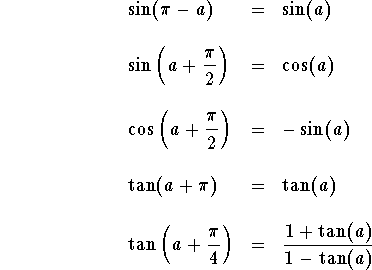
More identities may be proved similar to the above ones. The bottom line is to remember the addition formulas and use them whenever needed.
Double-Angle and Half-Angle Formulas
Double-Angle and Half-Angle formulas are very useful. For example, rational functions of sine and cosine will be very hard to integrate without these formulas. They are as follow

Example. Check the identities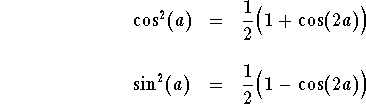 Answer. We will check the first one. the second one is left to the reader as an exercise. We have
Answer. We will check the first one. the second one is left to the reader as an exercise. We have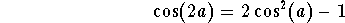 Hence
Hence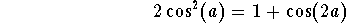 which implies
which implies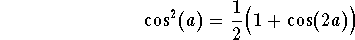 Many functions involving powers of sine and cosine are hard to integrate. The use of Double-Angle formulas help reduce the degree of difficulty.
Many functions involving powers of sine and cosine are hard to integrate. The use of Double-Angle formulas help reduce the degree of difficulty.
Example. Write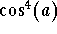 as an expression involving the trigonometric functions with their first power.Answer. We have
as an expression involving the trigonometric functions with their first power.Answer. We have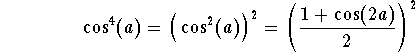 Hence
Hence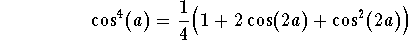 Since
Since , we get
, we get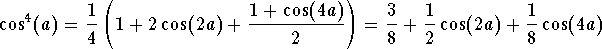 or
or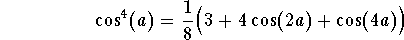
Example. Verify the identity Answer.We have
Answer.We have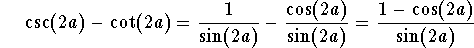 Using the Double-Angle formulas we get
Using the Double-Angle formulas we get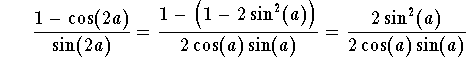 Putting stuff together we get
Putting stuff together we get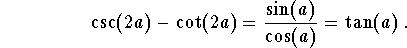 From the Double-Angle formulas, one may generate easily the Half-Angle formulas
From the Double-Angle formulas, one may generate easily the Half-Angle formulas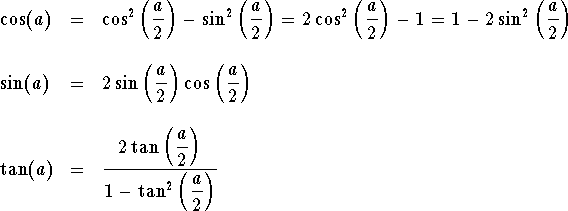 In particular, we have
In particular, we have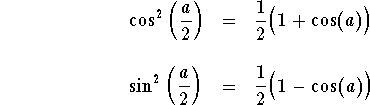
Example. Use the Half-Angle formulas to find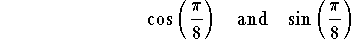 Answer. Set
Answer. Set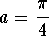 . Then
. Then Using the above formulas, we get
Using the above formulas, we get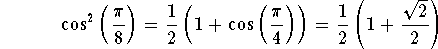 Since
Since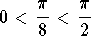 , then
, then 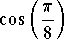 is a positive number. Therefore, we have
is a positive number. Therefore, we have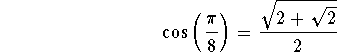 Same arguments lead to
Same arguments lead to
Example. Check the identities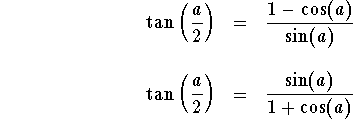 Answer. First note that
Answer. First note that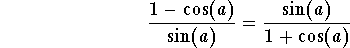 which falls from the identity
which falls from the identity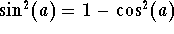 . So we need to verify only one identity. For example, let us verify that
. So we need to verify only one identity. For example, let us verify that using the Half-Angle formulas, we get
using the Half-Angle formulas, we get which reduces to
which reduces to
Product and Sum Formulas
From the Addition Formulas, we derive the following trigonometric formulas (or identities)

Remark. It is clear that the third formula and the fourth are identical (use the property to see it).The above formulas are important whenever need rises to transform the product of sine and cosine into a sum. This is a very useful idea in techniques of integration.
to see it).The above formulas are important whenever need rises to transform the product of sine and cosine into a sum. This is a very useful idea in techniques of integration.
Example. Express the product as a sum of trigonometric functions.Answer. We have
as a sum of trigonometric functions.Answer. We have which gives
which gives Note that the above formulas may be used to transform a sum into a product via the identities
Note that the above formulas may be used to transform a sum into a product via the identities
Example. Express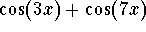 as a product.Answer. We have
as a product.Answer. We have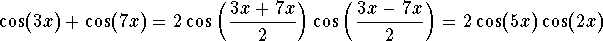 Note that we used
Note that we used .
.
Example. Verify the formula Answer. We have
Answer. We have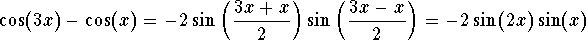 and
and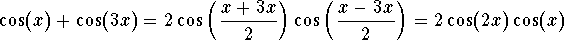 Hence
Hence which clearly implies
which clearly implies
Example. Find the real number x such that and
and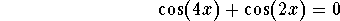 Answer. Many ways may be used to tackle this problem. Let us use the above formulas. We have
Answer. Many ways may be used to tackle this problem. Let us use the above formulas. We have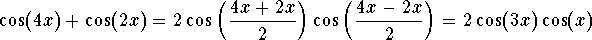 Hence
Hence Since
Since , the equation
, the equation 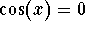 gives
gives 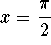 and the equation
and the equation  gives
gives  . Therefore, the solutions to the equation
. Therefore, the solutions to the equation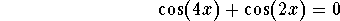 are
are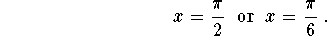
Example. Verify the identity Answer. We have
Answer. We have Using the above formulas we get
Using the above formulas we get Hence
Hence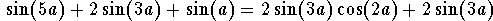 which implies
which implies Since
Since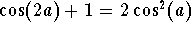 , we get
, we get
TRIGONOMETRIC EQUATIONS
Some equations which involve trigonometric functions of the unknown may be readily solved by using simple algebraic ideas (as Equation 1 below), while others may be impossible to solve exactly, only approximately (e.g., Equation 2 below):

EXAMPLE 1: Find all solutions of the equation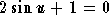 .Solution: We can graphically visualize all the angles u which satisfy the equation by noticing that
.Solution: We can graphically visualize all the angles u which satisfy the equation by noticing that is the y-coordinate of the point where the terminal side of the angle u (in standard position) intersects the unit circle (see Figure 1):We can see that there are two angles in
is the y-coordinate of the point where the terminal side of the angle u (in standard position) intersects the unit circle (see Figure 1):We can see that there are two angles in that satisfy the equation:
that satisfy the equation:  and
and 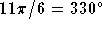 . Since the period of the sine function is
. Since the period of the sine function is  , it follows that all solutions of the original equation are:
, it follows that all solutions of the original equation are: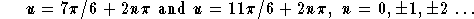
Table of Trigonometric Identities
Reciprocal identities

Pythagorean Identities
Quotient Identities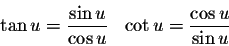
Co-Function Identities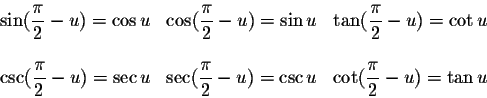
Even-Odd Identities
Sum-Difference Formulas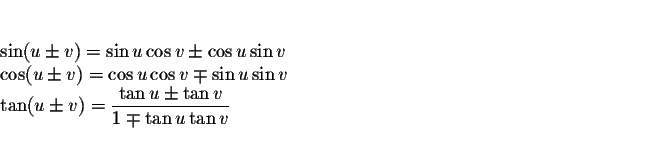
Double Angle Formulas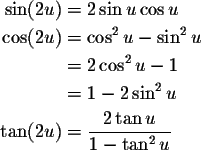
Power-Reducing/Half Angle Formulas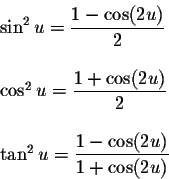
Sum-to-Product Formulas
Product-to-Sum Formulas
SOLVING TRIGONOMETRIC EQUATIONS
This sections illustrates the process of solving trigonometric equations of various forms. It also shows you how to check your answer three different ways: algebraically, graphically, and using the concept of equivalence.The following table is a partial lists of typical equations.
Solve for x in the following equations.
Example 1: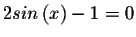
There are an infinite number of solutions to this problem. To solve for x, you must first isolate the sine term.
 We know that the
We know that the therefore
therefore  The sine function is positive in quadrants I and II. The
The sine function is positive in quadrants I and II. The  is also equal to
is also equal to  Therefore, two of the solutions to the problem are
Therefore, two of the solutions to the problem are  and
and 
The period of the sin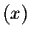 function is
function is 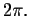 This means that the values will repeat every
This means that the values will repeat every 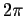 radians in both directions. Therefore, the exact solutions are
radians in both directions. Therefore, the exact solutions are  and
and 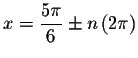 where n is an integer. The approximate solutions are
where n is an integer. The approximate solutions are 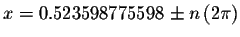 and
and 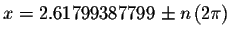 where n is an integer.
where n is an integer.
These solutions may or may not be the answers to the original problem. You much check them, either numerically or graphically, with the original equation.Numerical Check:Check answer .
- Left Side:

- Right Side: 0
Since the left side equals the right side when you substitute for x, then
for x, then  is a solution.
is a solution.
Check answer .
- Left Side:

- Right Side: 0
 for x, then
for x, then  is a solution.
is a solution.
Graphical Check:Graph the equationf (x) = 2 sin(x) - 1Note that the graph crosses the x-axis many times indicating many solutions. Note that it crosses at . Since the period is
. Since the period is  , it crosses again at 0.5236+6.283=6.81 and at0.5236+2(6.283)=13.09, etc. The graph crosses at
, it crosses again at 0.5236+6.283=6.81 and at0.5236+2(6.283)=13.09, etc. The graph crosses at  . Since the period is
. Since the period is  , it will cross again at 2.618+6.283=8.9011 and at 2.618+2(6.283)=15.18, etc.
, it will cross again at 2.618+6.283=8.9011 and at 2.618+2(6.283)=15.18, etc.
- Example 2:

There are an infinite number of solutions to this problem. To solve for x, you must first isolate the sine term. If we restriction the domain of the sine function to
If we restriction the domain of the sine function to![$\left[ -\displaystyle \frac{\pi }{2}
\leq 3x\leq ,\displaystyle \frac{\pi }{2}\...
...[ -\displaystyle \frac{\pi }{6}\leq
x\leq ,\displaystyle \frac{\pi }{6}\right] $](http://www.sosmath.com/algebra/solve/solve9/s92/img3.gif) , we can use the inverse sine function to solve for reference angle 3x and then x.
, we can use the inverse sine function to solve for reference angle 3x and then x. 
 We know that the
We know that the e function is positive in the first and the second quadrant. Therefore two of the solutions are the angle 3x that terminates in the first quadrant and the angle
e function is positive in the first and the second quadrant. Therefore two of the solutions are the angle 3x that terminates in the first quadrant and the angle  that terminates in the second quadrant. We have already solved for 3x.
that terminates in the second quadrant. We have already solved for 3x.
 The solutions are
The solutions are and
and  The period of the
The period of the function is
function is  This means that the values will repeat every
This means that the values will repeat every  radians in both directions. Therefore, the exact solutions are
radians in both directions. Therefore, the exact solutions are  and
and where n is an integer.
where n is an integer.
The approximate solutions are and
and  where n is an integer.
where n is an integer.
These solutions may or may not be the answers to the original problem. You much check them, either numerically or graphically, with the original equation.
Numerical Check:
Check the answer x=0.174532925
- Left Side:

- Right Side:

Since the left side equals the right side when you substitute 0.174532925for x, then 0.174532925 is a solution.
Check the answer x=0.872665
- Left Side:

- Right Side:

Since the left side equals the right side when you substitute for x, then
for x, then  is a solution.
is a solution.
Graphical Check:
Graph the equation Note that the graph crosses the x-axis many times indicating many solutions. You can see that the graph crosses at 0.174532925. Since the period is
Note that the graph crosses the x-axis many times indicating many solutions. You can see that the graph crosses at 0.174532925. Since the period is , it crosses again at0.174532925+2.094395=2.2689 and at 0.174532925+2(2.094395)=4.3633, etc. The graph crosses at 0.872665.
, it crosses again at0.174532925+2.094395=2.2689 and at 0.174532925+2(2.094395)=4.3633, etc. The graph crosses at 0.872665.
Since the period is , it will cross again at
, it will cross again at  and at 0.872665+2(2.094395)=5.061455, etc
and at 0.872665+2(2.094395)=5.061455, etc 
The Derivatives of Trigonometric Functions
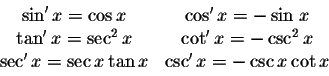 Trigonometric functions are useful in our practical lives in diverse areas such as astronomy, physics, surveying, carpentry etc. How can we find the derivatives of the trigonometric functions?Our starting point is the following limit:
Trigonometric functions are useful in our practical lives in diverse areas such as astronomy, physics, surveying, carpentry etc. How can we find the derivatives of the trigonometric functions?Our starting point is the following limit:
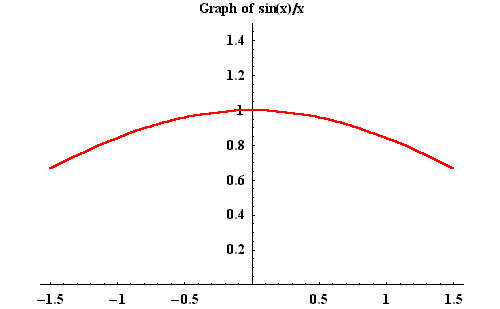 Using the derivative language, this limit means that
Using the derivative language, this limit means that . This limit may also be used to give a related one which is of equal importance:
. This limit may also be used to give a related one which is of equal importance: 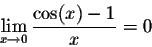
To see why, it is enough to rewrite the expression involving the cosine as
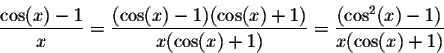
But , so we have
, so we have

This limit equals and thus
and thus  .
.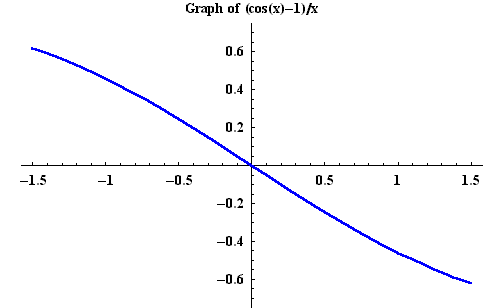 In fact, we may use these limits to find the derivative of
In fact, we may use these limits to find the derivative of and
and  at any point x=a. Indeed, using the addition formula for the sine function, we have
at any point x=a. Indeed, using the addition formula for the sine function, we have 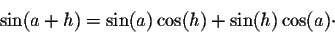
So
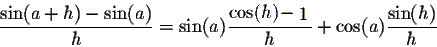
which implies
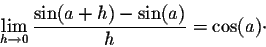
So we have proved that exists and
exists and  .Similarly, we obtain that
.Similarly, we obtain that exists and that
exists and that  .Since
.Since ,
,  ,
, 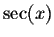 , and
, and  are all quotients of the functions
are all quotients of the functions  and
and  , we can compute their derivatives with the help of the quotient rule:
, we can compute their derivatives with the help of the quotient rule:
 It is quite interesting to see the close relationship between
It is quite interesting to see the close relationship between and
and 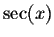 (and also between
(and also between  and
and  ).From the above results we get
).From the above results we get

These two results are very useful in solving some differential equations.
Example 1. Let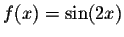 . Using the double angle formula for the sine function, we can rewrite
. Using the double angle formula for the sine function, we can rewrite 
So using the product rule, we get
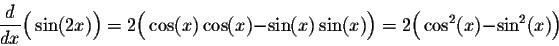
which implies, using trigonometric identities,
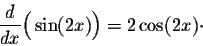
Hyperbolic Functions
The hyperbolic functions enjoy properties similar to the trigonometric functions; their definitions, though, are much more straightforward:
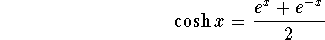
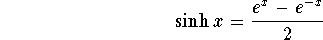
Here are their graphs: the (pronounce: "kosh") is pictured in red, the
(pronounce: "kosh") is pictured in red, the  function (rhymes with the "Grinch") is depicted in blue.
function (rhymes with the "Grinch") is depicted in blue.
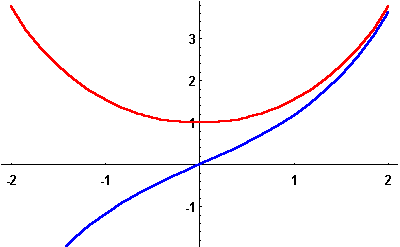
As their trigonometric counterparts, the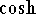 function is even, while the
function is even, while the  function is odd.
function is odd.
Their most important property is their version of the Pythagorean Theorem.
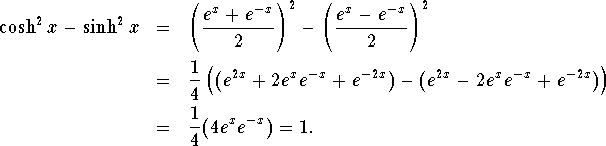
While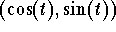 ,
, 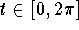 , parametrizes the unit circle, the hyperbolic functions
, parametrizes the unit circle, the hyperbolic functions 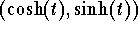 ,
, 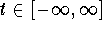 , parametrize the standard hyperbola
, parametrize the standard hyperbola  , x>1.
, x>1.
In the picture below, the standard hyperbola is depicted in red, while the point for various values of the parameter t is pictured in blue.
for various values of the parameter t is pictured in blue.

The other hyperbolic functions are defined the same way, the rest of the trigonometric functions is defined:

tanh x coth x 

sech x csch x 

For every formula for the trigonometric functions, there is a similar (not necessary identical) formula for the hyperbolic functions:
Let's consider for example the addition formula for the hyperbolic cosine function:

Inverse Hyperbolic Functions
The hyperbolic sine function is a one-to-one function, and thus has an inverse. As usual, we obtain the graph of the inverse hyperbolic sine function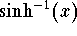 (also denoted by
(also denoted by  ) by reflecting the graph of
) by reflecting the graph of about the line y=x:
about the line y=x: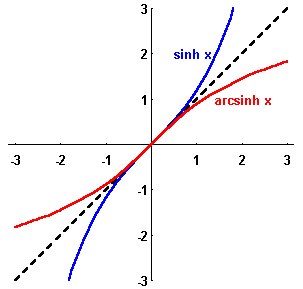 Since
Since is defined in terms of the exponential function, you should not be surprised that its inverse function can be expressed in terms of the logarithmic function:Let's set
is defined in terms of the exponential function, you should not be surprised that its inverse function can be expressed in terms of the logarithmic function:Let's set , and try to solve for x:
, and try to solve for x: This is a quadratic equation with
This is a quadratic equation with instead of x as the variable. y will be considered a constant.So using the quadratic formula, we obtain
instead of x as the variable. y will be considered a constant.So using the quadratic formula, we obtain Since
Since for all x, and since
for all x, and since  for all y, we have to discard the solution with the minus sign, so
for all y, we have to discard the solution with the minus sign, so and consequently
and consequently Read that last sentence again slowly!We have found out that
Read that last sentence again slowly!We have found out that
Here is some problems which have Trigonometry Solutions with detail explanation step by step .
Question 1
Question:
Answer:



Question 2
Question: Answer:
Answer:

Question 3
Question: Answer:
Answer:

Question 4
Question: Answer:
Answer:



Question 5
Question:
Answer:
Question 6
Question: If A+B=45, prove that (1 + tan A) (1 + tan B) = 2.
Answer:





Question 7
Question: Answer:
Answer:


Question 8
Question: Answer:
Answer:






Question 9
Question: Prove that tan 70o = tan 20o + 2 tan 50o.Answer:







Trigonometry Solutions:Question 10
Question: Answer: Here is the Trigonometry Solution , By data:
Answer: Here is the Trigonometry Solution , By data:

By componendo and dividendo

- Left Side:



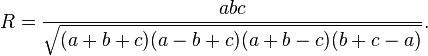
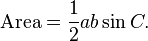
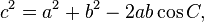

![\frac{a-b}{a+b}=\frac{\tan\left[\tfrac{1}{2}(A-B)\right]}{\tan\left[\tfrac{1}{2}(A+B)\right]}](http://upload.wikimedia.org/math/c/b/5/cb5c755b587d4e4e1f4367c492c4de8d.png)



3 comments:
I’m happy I located this blog! From time to time, students want to cognitive the keys of productive literary essays composing. Your first-class knowledge about this good post can become a proper basis for such people. nice one
https://trig properties
Check here SciPiPupil: Solutions To Every Academic Questions of Class 9 and Class 10. All these solutions are accurate and will definitely help you in the examinations of 9 and 10.
Excellent information you have shared, thanks for taking the time to share with us such a great article. I really appreciate your work. Also, click here for Trigonometry Formulas and Definitions or many more.
Post a Comment